Astronomers have relied on total solar eclipses since 1919 in order to carefully measure the positions of a few stars appearing near the Sun. Those locations differed from their positions when they were observed far from the Sun, showing typically 1 arcsecond deflections as predicted by Einstein’s General Theory of Relativity. Repeating this experiment to get better results always had a number of difficulties, including the requirements of setting up at an inconvenient location to get a few photographs, once every few years, and hoping the weather would not be cloudy or too windy. Successful expeditions were rare¹, and only in 2017 was the entire experiment finally completed without problems².

To repeat the experiment during the daytime without an eclipse was expected to be a challenge, but probably easier and less risky than travelling to an eclipse site. This was astronomer E. Finlay Freundlich’s suggestion, although Einstein was skeptical³. Astronomers in Great Britain were successful in imaging Capella and a few other bright stars 50° from the Sun in 1916, but never reported any further results⁴.

To complete this experiment using current technology includes four requirements. Each of these were addressed during the planning stages.

1. Appropriate target stars need to be identified,

2. The target stars need to be imaged within 4° of the Sun,

3. The location of the stars need to be measured with 0.02-pixel precision, and

4. The telescope plate scale needs to be measured to 3 part-per-million (PPM) accuracy.

If these four items could be demonstrated, then the experiment should succeed. This paper completed the first three items, but, in spite of a concerted effort to be described here, failed on the last one.

This paper explains the equipment and techniques followed for the four items. The procedure for identifying the target stars is outlined, and the equipment used to image them close to the Sun is presented. The automated software program to measure the star locations is reviewed with test data that demonstrates its accuracy. The final step, measuring the plate scale, was accomplished using artificial stars, and the cause for that failure is suggested. The paper concludes with the outline of two completely different approaches which were also considered, but in the final analysis, still had significant risks.

2. Selection of the Target stars.

Based on sky transparency measurements at various altitudes, it was once proposed that relatively dim stars could be seen near the sun without the need for a total solar eclipse⁵. For every km increase in elevation, the sky was measured 1.5 stellar magnitudes darker. On Mauna Kea (4200 m elevation) the advantage was expected to be an amazing 6.3 magnitudes compared to sea level, using photographic plates with a red filter. However, no further experiments have ever been reported.

In an attempt to verify this sky brightness change at various altitudes, a series of measurements were made several times at various locations around California, Arizona, Colorado, and Wyoming. A small CCD camera with an 852 nm (10 nm bandpass) filter was used with a long sun shield to prevent direct sunlight from entering the lens when pointed within 5° of the Sun. The results were not encouraging, showing only very slight (nominally 1 stellar magnitude) reductions in sky brightness even at 3300 m compared to sea level. Based on the old photographic tests, a factor of 100x reduction in sky brightness was expected. Local humidity or wind-blown particulates apparently caused a much larger sky background effect than altitude. However, under dry sky conditions at sea level, with enhanced baffling, the background was reduced enough to record SNR=20 measurements of stars down to approximately 3^rd magnitude, for stars further than about 4° from the sun, in single 0.3 sec exposures.

For stellar measurements, a wide camera angle to accommodate a 5° radius centered on the Sun was not practical, and would make the plate scale nominally 10 arcsec/pixel. This would make precise stellar location measurements impossible. Instead of measuring the absolute deflection of stars surrounding the sun, the differential deflection of a pair of stars could be more easily measured. The pair needed to be within the small field of view of a camera, so that limited the selection to one particularly bright pair that appears nearly radially oriented with respect to the Sun close to the ecliptic. This star pair could be measured during late June and again in early July. While their absolute deflections are on the order of 0.12 arcsec, the differential deflection over the closest measureable distance of 4° is near 0.04 arcsec. While these are small numbers, for a plate scale near 1.5 arcsec/pixel for a 2° field of view, the experiment seemed possible. Table 1 summarizes the target star features.

Table 1. Target star features.

	Star 1	Star 2
HIP ID	30343	29655
Magnitude	2.87	3.33
Spectral class	M0	M1
Separation (arcseconds)	6722
Distance from Sun during late June (degrees)	5.5 to 3.7	7.4 to 5.5
Distance from Sun during early July (degrees)	3.3 to 6.1	5.1 to 7.9
Typical SNR	20

3. Imaging the Target stars.

The target star pair is located at a high declination, so the experiment is best done in the Northern hemisphere. From San Diego, the pair reaches 80° altitude in the summer, good for minimizing both atmospheric turbulence and atmospheric refraction. In order to determine the centroid, a small diameter image is preferred, since a smaller diameter reduces the number of pixels used in the background noise calculations, increasing the SNR.

A wide assortment of telescopes and cameras were evaluated, with the optimum combination selected. Because the target star pair is separated by nearly 1.9°, the telescope optics needed to produce good images only at ± 0.85° off axis; field curvature was not important. Since the filter spectral band was only 10 nm, this eliminated chromatic errors. An old Orion doublet refractor telescope with a focal length of 500 mm was selected for the preliminary experiments, with the aim to replace it with a newer apochromatic refractor before the final experiment. An improvement in the off-axis images was realized by stopping the aperture from 80 mm down to 50 mm. There is plenty of signal light, but the tradeoff here was to not have diffraction contribute significantly to the seeing-limited image. The interior of the telescope, as well as the added external sun shade, was painted with latex paint measured to have a reflectivity of only 2.3% at 852 nm.

A CMOS camera was selected so that more images could be recorded in the nominal 4 hour period when the stars were at the highest elevation. The impact of a 12-bit readout compared to a 16-bit readout was shown to be negligible for a similar experiment². The sky background was so high that readout noise and dark noise were negligible. The ZWO ASI1600Pro TE-cooled camera⁶, with 3.8 um pixels and a diagonal of 22 mm, met the requirements for this experiment. The combination provided 1.57 arcsec per pixel. The best daytime target star images were 3 pixels FWHM. Since the diffraction limit was about 3.5 arcsec, or 2.3 pixels, turbulence contributed about half of the broadening.

Figure 1. The refractor has an extended sunshade attached to the front, and is mounted on a fixed pedestal. A piece of white cardboard shields the focuser from direct sunlight.

A small resistive heater bolted to the side of the telescope was cycled with a 3 minute period to slowly tilt the optical axis about 1.5 pixels peak-peak amplitude. This small shift moved the image to reduce pixel phase errors⁷.

The telescope was driven on a Bisque Paramount MyT equatorial mount⁸. This small mount is portable and rigidly handles the small refractor and camera. To reduce sky background as the Sun moved across the sky, a second equatorial mount was placed adjacent to the telescope. This mount held only a 20 cm x 25 cm cardboard piece held about 3 m from the telescope. Its shadow followed the imaging telescope aperture, blocking the Sun as close as 4° away. Wind slightly affected this shield, but the imaging telescope remained very stable, producing good stellar images.

To determine the plate scale, a stable internal autocollimator was preferred over using a second star pair. This doubles the target star imaging time and allows simultaneous calibrations for every image without having the uncertainty of telescope plate scale change for a different telescope pointing vector. An autocollimator was successfully designed and implemented to complete this experiment. The details of those optics is discussed in Section 5.

4. Determining the Star Location

Since tens of thousands of images were collected, an automatic procedure was necessary to determine the star locations on the focal plane. A Python program was written to accomplish this task. The separation of the target stars was about 6722 arcsec, or 4280 pixels. Since the measurement precision goal was nominally 0.02 arcsec, this amounts to about 3 parts-per-million (PPM). Additionally, 0.03 arcsec corresponds to 0.02 pixels. The software needed to calculate centroids to 0.01 pixel precision.

The procedure was to first average 10 seconds worth of images in each series, in order to reduce noise and improve the SNR. This included between 10 and 25 images, depending on the exposure time. This resulted in SNRs about 100 for the tests when the target stars were 45° from the Sun. During these 10 seconds, the mount tracking error was negligible, so the images were not re-centered before averaging.

The software then found the approximate centroid location using a simple moment calculation over a 14 pixel radius. The mean and RMS backgrounds were calculated over a 28 pixel inner radius centered over this initial estimate, using an annulus 12 pixels wide. Within this 14 pixel radius, every pixel count was reduced by a value equal to the background mean plus three times the background RMS, following the procedure developed by Stone⁹. This clips the background noise more than the signal, improving the final centroid calculated over a 7 pixel radius. This was repeated for every 10-second average over the multi-hour data acquisition period. This program output was compared to sampled results from the manually operated MaxIm DL program¹⁰. The results were similar, with random errors about 0.02 pixels.

Several small corrections would be necessary in the final experiment to calculate the accurate separation between the target stars, including the varying atmospheric parameters. The USNO program NOVAS can do those corrections automatically¹¹, but were not necessary in the preliminary tests.

5. Determining the accurate plate scale

This requirement turned out to be the most difficult part of the experiment. The first approach was to identify a pair of calibration stars with approximately the same spacing and orientation as the target pair, but further from the Sun. This is difficult, but possible, because the large area of the sky in which to search. The main drawback is that the plate scale might change as the telescope is re-pointed to the calibration pair, and this also subtracts half of the time that could be used to image the target pair. An alternative approach was chosen; designing and implementing an artificial star pair that is imaged simultaneously with every target star image.

This optical design was based on using reflections from both surfaces of an N-BK7 glass wedge placed just in front of the telescope aperture. The uncoated wedge had a mechanical angle near 0.5°, providing two reflections that were 1.562° apart. An infrared LED placed behind a 10 micron pinhole acted as an artificial star near the focal plane. A small right angle prism attached to the 852 nm filter near the focal plane reflected the light toward the aperture, where the light was collimated and reflected back to the camera. The prism blocked some of the focal plane, but that did not affect the analysis, since the target stars and the artificial stars were imaged near the edges of the CMOS sensor. Since the filter bandwidth was only 10 nm, chromatic dispersion for this small wedge angle was negligible for both the artificial and the target stars.

Figure 2. A 300 pixel x 4656 pixel ROI was used to increase frame rate and save memory space. The central portion was blocked by the prism. All star locations are circled. The outer two are the target stars and the other four are the artificial stars.

To provide an accurate measurement of the calibration angle stability produced by the wedge, a second optical wedge was placed just in front of the first wedge. This provided a second pair of artificial stars with a slightly different angle, but the ratio of the two pairs of angles should be constant. Since stability of these artificial star pairs was crucial to generating an accurate plate scale, both wedges were wrapped along their edges with several layers of copper braid on top of thermal grease, to minimize any potential thermal gradients.

Figure 3. The two optical wedges were wrapped with tinned copper braid to reduce potential thermal gradients across the diameter. A layer of thermal grease was added before the braid was wound around the edges.

This design appeared to be the ideal solution. The two reflections from each wedge should be very stable over temperature and small focal length changes, giving a fixed calibration to measure the plate scale. Tests done indoors with a fixed vertical orientation did show a stability on the order of 1 PPM, adequate for the experiment. Unfortunately, outdoor tests with the telescope tracking the sky did not show the same stability.

Figure 4. For an indoor test, after a one hour period, the plate scale ratio was stable to a few PPM.

6. Data analysis

By comparing the ratio of the artificial star separations, the stability of the plate scale was continuously monitored for several hours, and this test was repeated over several days. The ratio changed randomly on the order of 10 PPM to 30 PPM, much larger than expected. This same error would be propagated to the target stars. The artificial stars had a large SNR, and their brightness was adjusted to about 60% of saturation. The SNR for the target stars was also high, because these tests were done in August, when the Sun was about 45° away. The centroid noise could be reduced to the few PPM level by averaging over thousands of images, but it was clear there was some slow random change that far exceeded the experimental requirement.

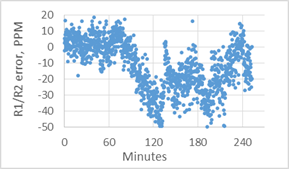
Figure 5. For an outdoor test with tracking, after a good start, the plate scale wandered more than 30 PPM, uncorrelated to temperature or star position.

Tests were done both during stable and rising temperatures, with the telescope both static and tracking. The location of the artificial stars wandered unidirectionally over dozens of pixels due to the mechanical effects of tilt and increasing temperature. The telescope’s optical distortion was estimated from some nighttime measurements, and should be negligible for these small changes in the optical alignment. Changes in the wedge reflection angle ratios would require very high temperature changes; this was not the case.

The only remaining cause of the plate scale change must be due to the focal plane characteristics. The optical filter, camera window, and CMOS sensor window may have small ripples in the glass that cause very small changes in the image location, even though they are close to the sensor. The center distances of camera pixels was investigated for a large CCD camera while the URAT1 star catalog was being assembled, and random errors larger than this were seen across the focal plane¹². The warping distortion for that camera was stable, as it was mapped using thousands of stars, so it was correctable. In this experiment, the requirement is much higher, so a mapping over individual pixels would be required. If the telescope tracking were stable enough and the telescope hardware was rigid enough and temperature controlled with additional thermal insulation, then this correcting for this effect might work. That would be well beyond the scope of this project, but might make the experiment possible for some other dedicated astronomer.

7. Conclusions

The baseline configuration presented here seemed to be the most stable design, but unknown errors prevented a successful experiment. Two other experimental configurations are suggested here that might be considered in a future implementation. They have a different set of potential problems, but might be successful.

The main problem here was that the stars were too far apart on the focal plane. If optics are added to prevent this, then some of the problems should disappear. It is important that the optics are always common-path, to avoid generating new errors. For the first example, an achromatic optical wedge can be placed near the refractor aperture. Starlight from one target star could be reflected from one surface, while the starlight from the second target star could be reflected from the other surface. This is not very photon-efficient, but there is plenty of light from the stars, and the background light behind each target star would only be doubled. The reflective wedge angle should be almost identical to the target star separation, so that the two stars are separated by only a few pixels on the focal plane. The differential measurement of the star centroids would be subject to fewer errors.

Another design would be to make absolute star position measurements instead of differential measurements. This increases the angular deflections by a factor of three. A portion of the Sun’s disk could be imaged to provide both a plate scale reference and distance reference. Common-path requirements would again require an achromatic wedge prism. One side would reflect the Sun’s disk, while the other side reflects the two target stars. This technique would still require the same target star pair used here, but since the wedge angle would have to be fixed, the experiment would only operate for a single hour as the Sun moves to the correct position.

The successful completion of this project would only be of historical interest, since this is how 20^th century astronomers originally suggested the solar deflection be measured. Unfortunately, this is not likely to happen from observatories on the ground. It was disappointing to abandon this experiment, especially since the root cause of the plate scale drift is only suspected. However, the standard technique of using total solar eclipses to measure the deflection can still be improved upon. There are a number of convenient, long total eclipses coming up in the next two decades and they should offer opportunities for interested astronomers for additional attempts at repeating the experiment that made Einstein famous.

8. Acknowledgement

The author thanks Stan Moore for helpful discussions during the operation of this experiment, and for suggesting one of the alternate design approaches.

9. References

1. von Kluber, H., “The Determination of Einstein’s Light Deflection in the Gravitational Field of the Sun”, Vistas in Astronomy 3, pp.47-77 (1960).

2. Bruns, D.G., “Gravitational Starlight Deflection Measurements During the 21 August 2017 Total Solar Eclipse”, Class. Quantum Grav. 35, #7, 075009 (2018).

3. Crelinsten, J. “Einstein’s Jury”, p.56, Princeton University Press (2006).

4. A. F. Lindemann and F. A. Lindemann, “Daylight Photography of Stars as a Means of Testing the

Equivalence Postulate in the Theory of Relativity,” MNRAS 77, pp.140-151 (1916).

5. Handler, F.A. and R.A. Matzner, “Photographic Astrometry against a Bright Sky: Theory and Application”, AJ 83 No.10, pp.1227-1234 (1978).

6. ZWO web site: www.astronomy-imaging-camera.com

7. Anderson, J, and I. R. King, “Toward High-precision Astrometry with WFPC2. I. Deriving an Accurate Point-spread Function”, PASP 112, #776, pp.1360-1382 (2000).

8. Bisque web site: www.bisque.com

9. Stone, R.A., “A comparison of Digital Centering Algorithms”, AJ 97 #4, pp.1227-1237 (1989).

10. MaxIm DL web site: www.diffractionlimited.com

11. NOVAS website: aa.usno.navy.mil/software/novas/novas_info.php

12. N. Zacharias, C. Finch, J. Subasavage,

G. Bredthauer, C. Crockett, M. Divittorio, E. Ferguson, F. Harris, H. Harris,

A. Henden, C. Kilian, J. Munn, T. Rafferty, A. Rhodes, M. Schultheiss, T. Tilleman, and G. Wieder, “The First U.S. Naval Observatory Robotic Astrometric Telescope Catalog (URAT1)”, AJ 150, #4, (2015).

Thank you for your interest in Stellar Products!

Web page last updated March 24, 2021.